Physical changes such as state change and dissolving are reversible, and there is no change in total mass when they happen. Flowing and diffusion happens in liquids and gases, but not in solids.
The Conservation of Mass
Particles
All substances are made from particles. The arrangement, movement and closeness of these particles explain many of their properties.
Solids
Solids have a fixed shape and cannot flow because their particles are only able to vibrate about a fixed position. They cannot move from place to place.
Liquids
Liquids can flow and take the shape of the bottom of their container because their particles can move over each other. For example, when water is poured into a glass, the particles of water move over each other and into the corners of the glass. The particles keep on moving over each other as the water takes the shape of the glass.
Gases
Gases can flow to fill their container and to take its shape. This is because their particles can move in all directions. For example, when a Bunsen burner is connected to a gas tap and turned on, natural gas flows through the rubber tubing. The particles of natural gas are free to move anywhere inside the tubing, and pressure forces them through the tubing and into the Bunsen burner.
Density
Sometimes a scientist or an engineer needs to compare two or more materials. It must be a fair comparison and so the scientist or engineer will compare equal volumes of the materials. They might choose to compare 1 \(cm^3\) or 1 \(m^3\) of each material.
The mass of 1 \(cm^3\) or 1 \(m^3\) of a material is called its density.
The density of aluminium is 2.7 g/\(cm^3\). This means that a 1 \(cm^3\) block of aluminium has a mass of 2.7 g.
Definition
Density is the mass of 1\(cm^3\) or 1\(m^3\) of a material.
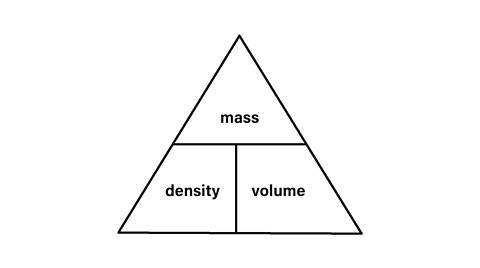
Formula
Density is calculated using the formula
Density =\(\frac{mass}{volume}\)

Unit
If mass is measured in grams, g, and volume in cubic centimetres, \(cm^3\), the unit of density is g/\(cm^3\).
If mass is measured in kilograms, kg, and volume in cubic metres, \(m^3\), the unit of density is kg/\(m^3\).
Density of gases, liquids and solids
Gases are much less dense than liquids because the particles of a gas are much further apart. 1 g of a gas will have much greater volume than 1 g of its liquid and so the density of the gas is much smaller.
The density of liquid oxygen = 1.1 g/\(cm^3\).
The density of oxygen gas = 0.0014 g/\(cm^3\).
Liquids are normally slightly less dense than solids because the particles of a liquid are slightly further apart. 1 g of a liquid will have slightly greater volume than 1 g of its solid and so the density of the liquid is slightly smaller.
The density of solid iron = 7.8 g/\(cm^3\).
The density of liquid iron = 6.9 g/\(cm^3\).
Measuring volume
To calculate density, the volume of the material must be known.
If the object is a regular shape, the volume can be found by measuring length, breadth and height and using the equation:Volume = length x breadth x height
If the object has an irregular shape, the volume can be measured using a measuring cylinder or a displacement can.
Volume of stone = final Volume ÔÇô initial Volume.
Volume of stone = 75 \(cm^3\) ÔÇô 50 \(cm^3\) = 25 \(cm^3\).
If the mass of the stone is measured using a top pan balance and found to be 175 g:
density =\(\frac{mass}{volume}\)
mass = 175 g
volume = 25 \(cm^3\)
density =\(\frac{175}{25}\)
density = 7 g/\(cm^3\)
The density of the stone is 7 g/\(cm^3\).
The volume of larger irregular objects can be measured using a displacement can.
- Fill the displacement can until the water is level with the bottom of the pipe.
- Place a measuring cylinder under the pipe ready to collect the displaced water.
- Carefully drop the stone into the can and wait until no more water runs into the cylinder.
- Measure the volume of the displaced water.
Experiments to measure density
Finding the density of a regular solid
Apparatus
Six regular objects of different materials, a 30 cm ruler, a top pan balance.
Method
Select the smallest object. Measure the length, breadth and height using a 30 cm ruler.Record the results in cm in a suitable table.
Calculate the volume of the object using: volume = length x breadth x height.
Record the volume in \(cm^3\) in the table.
Place the object on the top pan balance.
Record the mass in g in the table.
Repeat the procedure for the other five objects.
Calculate the density of each object using: density =\(\frac{mass}{volume}\).
Results
| Material | length / cm | breadth / cm | height / cm | Volume / \(cm^3\) | Mass / g | Density / (g/\(cm^3\)) |
|---|---|---|---|---|---|---|
Conclusion
Objects made of different materials have different densities.
Finding the density of an irregular shaped object
Apparatus
Five irregular objects of different materials, a measuring cylinder, a top pan balance.
Method
Select the smallest object and place it on the top pan balance.Record the mass in g in a suitable table.
Fill the measuring cylinder to the 50 \(cm^3\) mark. Record this volume.
Completely submerge the object in the water. Read and record the new volume of the water.
To calculate the volume of the object, subtract the original volume of water from the volume of the water plus object. Record the volume in the table.
Repeat the procedure for the other four objects.
Calculate the density of each object using: density =\(\frac{mass}{volume}\).
Results
| Object | Mass / g | Volume of water in measuring cylinder / \(cm^3\) | Volume of water + object / g | Volume of object / \(cm^3\) | Density / g/\(cm^3\) |
|---|---|---|---|---|---|
Finding the density of a liquid
Apparatus
A measuring cylinder, a top pan balance, tap water.
Method
Place an empty, dry measuring cylinder on the top pan balance. Read the mass and record in a suitable table in grams, g.
Remove the cylinder from the top pan balance. Pour 50\(cm^3\) of water into it and place it on the balance again. Read the mass and record in the table.
To calculate the mass of the water, subtract the mass of the empty cylinder from the mass of the cylinder plus water. Record the mass in the table.
Read the volume of water from the measuring cylinder. Record the volume of water in \(cm^3\).
Calculate the density of the water using: density =\(\frac{mass}{volume}\).
Repeat for a number of different liquids.
Results
| Liquid | Mass of empty measuring cylinder / g | Mass of measuring cylinder + liquid / g | Mass of liquid / g | Volume of liquid / \(cm^3\) | Density / g/\(cm^3\) |
|---|---|---|---|---|---|
| cold water | |||||
| hot water | |||||
| salt water | |||||
| olive oil |
Safety
Water should not be poured into the measuring cylinder when it is on the top pan balance.
Water spilled on the electric balance could cause electric shock.
Always remove the measuring cylinder from the balance before adding water.
Take care when pouring hot water.
Conclusion
The density of water is 1 g/\(cm^3\). In other words, 1\(cm^3\) of water has a mass of 1g.
Hot water is less dense than cold water.
Finding the density of air
Apparatus
Balloon, pump, top pan balance, clear container large enough to hold the inflated balloon
Method
Place an empty balloon on the top pan balance. Read the mass and record in a suitable table in grams, g.
Remove the balloon and using the pump, blow the balloon up fully. Tie a knot in the neck of the balloon. Place it on the balance again. Read the mass and record in the table.
To calculate the mass of air in the balloon, subtract the mass of the empty balloon from the mass of the balloon plus air. Record the mass in the table.
Mark the level of water in the clear container. Completely submerge the balloon in the water. Mark the new level of the water and remove the balloon.
Use a measuring cylinder to measure the amount of water needed to fill the container back up to the mark. The volume of water added is equal to the volume of the balloon. Record this value in the table.
Calculate the density of air using: density = m/v (g/\(cm^3\))
| Gas | Mass of empty balloon / g | Mass of balloon + air / g | Mass of air / g | Volume of balloon / \(cm^3\) | Density / (g/\(cm^3\)) |
|---|---|---|---|---|---|
| air |
ConclusionThe density of air is 0.0012 g/\(cm^3\).
1 \(cm^3\) of air has a mass of 0.0012 g

Density calculations
Density can be calculated using the equation:
density =\(\frac{mass}{volume}\)

There are three ways of arranging the density formula:
density =\(\frac{mass}{volume}\)
mass = volume x density
volume =\(\frac{mass}{density}\)
For density in g/\(cm^3\)
m = mass in g
v = volume in \(cm^3\)
For density in kg/\(m^3\)
m = mass in kg
v = volume in \(m^3\)
Example
1. What is the density of a material if 450 \(cm^3\) of it has a mass of 200 g?
mass = 200 g
volume = 450 \(cm^3\)
density =\(\frac{mass}{volume}\)
density = \(\frac{200}{450}\)
density 0.44 g/\(cm^3\)
The density of the material is 0.44 g/\(cm^3\).
2. A block of aluminium has dimensions: length = 5cm, breadth = 5cm, height = 2cm.
- Calculate the volume of the aluminium block.
- If its mass is 135g, find the density of aluminium.
i) volume = length x breadth x heightv = 5 x 5 x 2v = 50 \(cm^3\)
The volume of the aluminium block is 50 \(cm^3\).
ii) mass = 135 g
volume = 50 \(cm^3\)
density = \(\frac{mass}{volume}\)
density = \(\frac{135}{50}\)
density 2.7 g/\(cm^3\)
The density of the aluminium is 2.7 g/\(cm^3\).
3. Water has a density of 1 g/\(cm^3\). Calculate the mass of water in a 250\(cm^3\) measuring cylinder.
mass = ?
volume = 250 \(cm^3\)
Density = 1 g/\(cm^3\)
mass = volume x density
mass = 250 x 1mass = 250 g
The mass of water in the measuring cylinder is 250 g
4. Iron has a density of 7.8 g/\(cm^3\).
- What does that mean?
- Calculate the volume of 300 g of lead.
i) It means that 1 \(cm^3\) of lead has a mass of 7.8 g.
ii) mass = 300 g
volume = ?
density = 7.8 g/\(cm^3\)
volume =\(\frac{mass}{density}\)
volume =\(\frac{300}{7.8}\)
volume = 38.5 \(cm^3\)
The volume of lead is 38.5 \(cm^3\).
Floating and sinking

It is not true to say a stone sinks in water because it is heavier than water! A stone will sink in the Irish Sea ÔÇô but it is lighter than the whole of the Irish Sea!
Sinking and floating has to do with density!
An object or a liquid will float if it is less dense than the liquid beneath it.
Ice floats on top of water because the density of ice (0.9 g/\(cm^3\)) is less than the density of water (1.0 g/\(cm^3\)).

Most ships are made of steel which is a lot denser than water. If a block of steel is dropped into water it will sink, but a ship made of steel floats.
This is because the average density of the ship is less than that of water.
A lot of the volume of a ship is just air and so the average density of the ship (steel from which it is made, cargo, people and air contained within it) is less than the density of water, and so it floats.

Oil is less dense than water, and so oil floats on top of water.
Hot water rises and floats on top of cold water because hot water is less dense than cold water.
Hot air rises because it is less dense than the surrounding cold air.

More on Physics
Find out more by working through a topic
- count5 of 8

- count6 of 8

- count7 of 8

- count8 of 8
