Key points
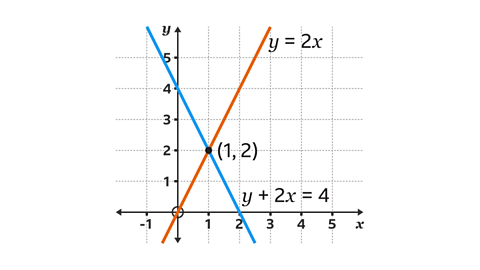
- An equationA mathematical statement showing that two expressions are equal. The expressions are linked with the symbol = is like a set of scales that are in balance. The termAn element within an algebraic sentence. Elements (terms) are separated by + or - signs. on either side of the equals sign (=) have the same value as each other.
- Equations need to stay in balance. Do the same thing to both sides to keep the equation balanced, for example adding or subtracting the same amount from each side.
- When a variableAn unknown value, usually represented by a letter like рќ’™ or рќ’љ is unknownA number that we do not know. They are commonly used in algebra, and sometimes referred to as variable and represented by symbols such as рќ’™ or рќ’љ it is often given a value of \(x\), although it is important to remember that any letter can be used to represent an unknown value.
- The unknown variable (often the letter \(x\)) can take any value, including decimal and negative values.

Solving equations when both unknown terms are positive
- If both unknown variables are positive, the first step is to subtract one of these \(x\) terms.
- Subtracting the \(x\) term which has the smaller coefficientA number or symbol multiplied with a variable or an unknown quantity in an algebraic term. Eg, 5 is the coefficient of 5рќ’Џ means that negative terms can be avoided.
- The \(x\) term with the smallest coefficient can appear on the left or the right of the = sign.
- This will reduce the number of terms and lead to a new equationA mathematical statement showing that two expressions are equal. The expressions are linked with the symbol = (still balanced) with just one unknownA number that we do not know. They are commonly used in algebra, and sometimes referred to as variable and represented by symbols such as рќ’™ or рќ’љ variable.
- Check the solution by substituting the final answer back into the original equation.
Example
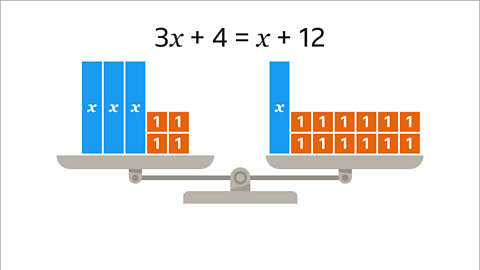
Image caption, The equation 3рќ’™ + 4 = рќ’™ + 12 can be represented by the diagram above. The equals sign between the two sets of terms shows that the equation is in balance.
Image caption, Both unknown variables (рќ’™ terms) are positive. The рќ’™ term with the smallest coefficient is identified. In this case it is the рќ’™ (or 1рќ’™) on the right-hand side of the equation.
Image caption, Subtract 1рќ’™ (usually written as рќ’™) from both sides of the equation to keep the equation in balance. This reduces the number of terms and produces a new equation of 2рќ’™ + 4 = 12
Image caption, There are unknown values (2рќ’™) and a number (4) on the left-hand side of the equation. The next step is to remove the number (4) from the left-hand side. Remember to keep the equation in balance by doing the same thing to the right-hand side of the equation.
Image caption, Subtract 4 from both sides of the equation. This creates a new equation of 2рќ’™ = 8
Image caption, Both sides are now divided by 2, which is the coefficient in front of the рќ’™ term in the original equation (2рќ’™). This reveals that рќ’™ = 4. If two lots of рќ’™ is equal to 8, then рќ’™ must equal 4
Image caption, It is important to check the answer. The original equation stated that 3рќ’™ + 4 = рќ’™ + 12. Substitute the value of 4 in place of the рќ’™ term.
Image caption, The solution is рќ’™ = 4. 4 multiplied by 3 plus 4 = 16 and one lot of 4 plus 12 = 16. When рќ’™ has a value of 4 then both sides of the equation are equal. This confirms the solution that рќ’™ = 4
1 of 8
Question
Find the value of the unknown term \(x\) when 2\(x\) + 5 = \(x\) + 8
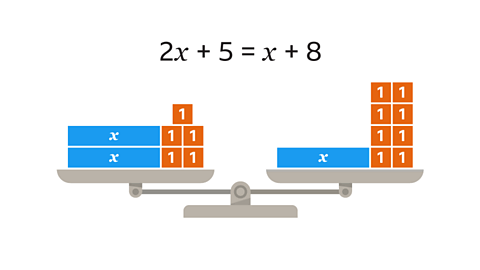
- Subtract an \(x\) term from each side to leave \(x\) + 5 = 8
- Subtract 5 from each side.
This gives the solution that \(x\) = 3

Solving equations when one unknown term is negative
- If one unknown variableAn unknown value, usually represented by a letter like рќ’™ or рќ’љ is negative, the first step is to add one of the \(x\) .
- Adding an \(x\) term helps avoid unknown values with negative coefficientA number or symbol multiplied with a variable or an unknown quantity in an algebraic term. Eg, 5 is the coefficient of 5рќ’Џ.
- The \(x\) term with a negative coefficient can appear on the left or the right of the equals sign.
- This will reduce the number of terms and lead to a new equation that is still in balance, with just one unknown variable.
Example
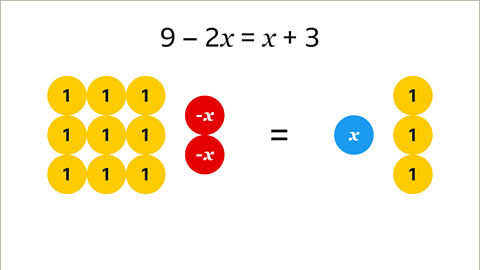
Image caption, The equation 9 - 2рќ’™ = рќ’™ + 3 can be represented by the diagram above. The equals sign between the two sets of terms shows that the equation is in balance.
Image caption, One unknown variable (рќ’™ term) is negative. Identify the рќ’™ term with the negative coefficient, which in this case is the -2рќ’™ on the left-hand side of the equation.
Image caption, Add 2рќ’™ to both sides of the equation to keep the equation in balance. This produces a new equation of 9 - 2рќ’™ + 2рќ’™ = 3рќ’™ + 3
Image caption, The positive 2рќ’™ and negative 2рќ’™ terms on the left-hand side of the equation cancel one another out. This now simplifies the equation to 9 = 3рќ’™ + 3
Image caption, There are unknown values (3рќ’™) and a number (3) on the right-hand side of the equation. The next step is to remove the number (3) from the right-hand side. Remember to keep the equation in balance by doing the same thing to the left-hand side of the equation.
Image caption, Subtract 3 from both sides of the equation. This creates a new equation of 6 = 3рќ’™
Image caption, If рќ’™ multiplied by 3 is equal to 6, рќ’™ must equal 2 (2 x 3 = 6). Both sides have been divided by 3 (the coefficient in front of the рќ’™ term) so рќ’™ = 2
Image caption, It is important to check the answer. The original equation stated that 9 – 2𝒙 = 𝒙 + 3. Substitute the value of 2 in place of the 𝒙 term.
Image caption, If 𝒙 = 2 then 9 – 2𝒙 = 9 – (2 x 2) = 5, and 𝒙 + 3 = 2 + 3 = 5. When 𝒙 is 2 both sides of the equation are equal. This confirms the solution that 𝒙 = 2
1 of 9
Question
Find the value of the unknown term \(x\) when \(x\) + 5 = 17 – 3\(x\)
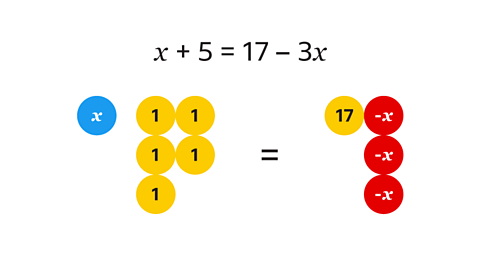
- Add 3\(x\) to each side to leave 4\(x\) + 5 = 17
- Subtract 5 from each side, resulting in 4\(x\) = 12
- Divide both sides of the equation by 4
This gives the solution that \(x\) = 3
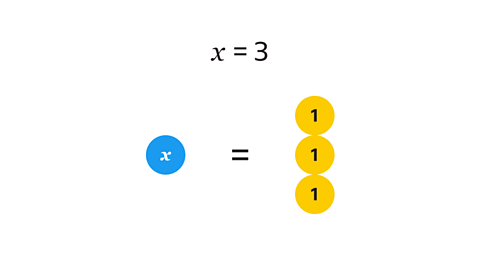
Practise solving equations with рќ’™ on both sides
Quiz
Practise solving equations with \(x\) on both sides with this quiz. You may need a pen and paper to help you with your answers.
Game - Divided Islands
Play the Divided Islands game! gamePlay the Divided Islands game!
Using your maths skills, help to build bridges and bring light back to the islands in this free game from ґуПуґ«ГЅ Bitesize.

More on Equations
Find out more by working through a topic
- count3 of 7
- count4 of 7

- count5 of 7

- count6 of 7